ELEC 226, Spring 2011
Prof. Rich Kozick

Homework 7

Date Assigned: Thursday, April 14, 2011
Date Due: Thursday, April 21, 2011 in class at 8:30 AM

1. Reading: In the Ulaby/Maharbiz text, please continue to study Chapter 5, sections 1 through 7. Please also read Chapter 6 and review your notes from MATH 212 on second-order, linear differential equations. We will not have time to study Chapter 6 in detail, but please browse the chapter for the main ideas.
   
   
2. Lab: We will have the final lab meeting on April 18 and 20, with no lab on April 25 and 27. We will finish Lab 6 on April 18 and 20, where you will demonstrate your waveform generator that produces square, triangle, and sine waves with variable amplitude and variable frequency.
   
   
3. Please solve the following problems in Chapter 5: Problems 33, 35, 38, 45, and 56.
   (Note that Problems 33 and 45 are the same circuits as Problems 34 and 46 on Homework 6, so you may want to solve these first.)
   
   
4. The following circuit is a slightly more general version of the clock circuit from Lab 5, where R_a and R_b may be unequal. Assume that the op amp is powered so that -10 V <= v_out(t) <= +10 V, and let T denote the period of the waveform produced by the oscillator circuit.

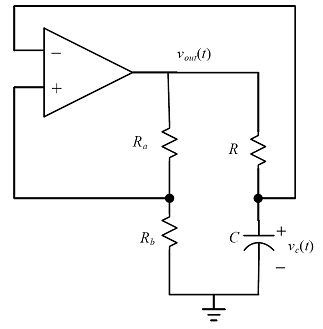
• Find the formulas for the capacitor voltage, v_c(t), during the time intervals 0 <= t <= T/2 and T/2 <= t <= T. Assume that the circuit is in "steady-state" at time t=0, i.e., the circuit has been oscillating for a long time before t=0. Also assume that the capacitor voltage has its minimum (most negative) value at t=0 (as we did in class on April 14).
  Your formulas will be a function of all of the resistor and capacitor values in the circuit (R, C, R_a, R_b).
• Use your results for v_c(t) to find a formula for the period, T, as a function of the resistor and capacitor values in the circuit.
• Sketch v_out(t) and v_c(t) versus time, and indicate the effects of the circuit parameters on the amplitudes and period in your plot.
• How should R_a and R_b be chosen so that v_c(t) is close to a triangle wave? Please explain based on your formulas for v_c(t).
  
  

Thank you.